Page 58 - Year 10 Knowledge Organiser

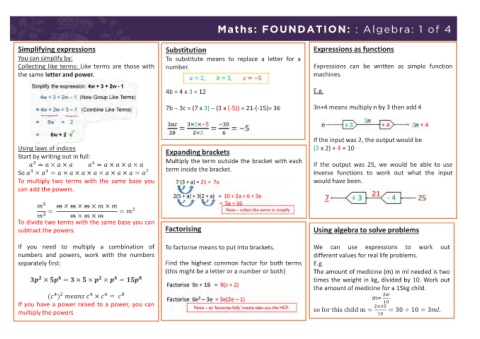
Maths: FOUNDATION: : Algebra: 1 of 4

Simplifying expressions Substitution Expressions as functions
You can simplify by: To substitute means to replace a letter for a
Collecting like terms: Like terms are those with number. Expressions can be written as simple function
the same letter and power. machines.

4b = 4 x 3 = 12 E.g.

7b – 3c = (7 x 3) – (3 x (-5)) = 21-(-15)= 36 3n+4 means multiply n by 3 then add 4

If the input was 2, the output would be
Using laws of indices Expanding brackets (3 x 2) + 4 = 10
Start by writing out in full:
3 4 Multiply the term outside the bracket with each If the output was 25, we would be able to use
= × × = × × × term inside the bracket.
3 4 7 inverse functions to work out what the input
So × = × × × × × × =
To multiply two terms with the same base you would have been.
can add the powers.

5
× × × × 2
= =
3 × ×
To divide two terms with the same base you can
subtract the powers. Factorising Using algebra to solve problems

If you need to multiply a combination of To factorise means to put into brackets. We can use expressions to work out
numbers and powers, work with the numbers different values for real life problems.
separately first: Find the highest common factor for both terms E.g.
(this might be a letter or a number or both) The amount of medicine (m) in ml needed is two

× = × × × = times the weight in kg, divided by 10. Work out
the amount of medicine for a 15kg child.
4 2
4
4
( ) × = 8 m= 2
If you have a power raised to a power, you can 2×15
10
multiply the powers so for this child m = 10 = 30 ÷ 10 = 3 .