Page 47 - Year 9 Knowledge Organiser

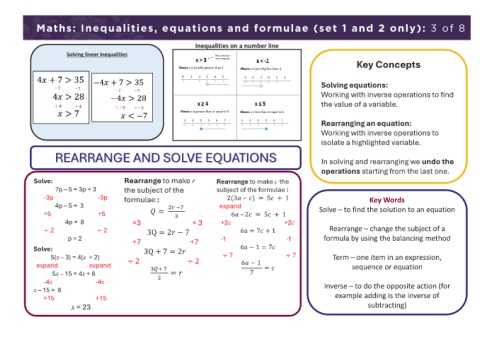
Maths: Quadratics(set 1 and 2 only): 2 of 8 Maths: Inequalities, equations and formulae (set 1 and 2 only): 3 of 8

Inequalities on a number line
Basic Algebra Expanding
Substitution Expanding Single Brackets Solving linear inequalities
Key Concepts
When we substitute values into a
Algebraic Indices Expanding double brackets that
Simplify formula we take out the variables look like single brackets Solving equations:
and put in the numbers.
You must use the rules of indices when simplifying Working with inverse operations to find
with algebra
Example: 2a + 4b the value of a variable.
Where a = -3 and b = 5
Rearranging an equation:
You do 2 x –3 = -6 Working with inverse operations to
And 4 x 5 = 20 Expanding double brackets
Solving Equations isolate a highlighted variable.
Then add them together: REARRANGE AND SOLVE EQUATIONS
-6 + 20 = 14 In solving and rearranging we undo the
operations starting from the last one.
When expanding brackets it Solve: Rearrange to make r Rearrange to make c the
is easier to use grid method. 7p – 5 = 3p + 3 the subject of the subject of the formulae :
Make sure you simplify at -3p -3p
2(3 – ) = 5 + 1
the end 4p – 5 = 3 formulae : expand Key Words
+5 +5 = 2 −7 6 – 2 = 5 + 1 Solve – to find the solution to an equation
3
4p = 8 ×3 × 3 +2 +2 Rearrange – change the subject of a
Quadratic Sequence ÷ 2 ÷ 2 3Q = 2 − 7 -1 -1 formula by using the balancing method
6a = 7 + 1
p = 2
Factorising Double Brackets Factorising into a single bracket Solve: +7 +7 6a − 1 = 7
Variables and numbers 5(x – 3) = 4(x + 2) 3Q + 7 = 2 ÷ 7 ÷ 7 Term – one item in an expression,
expand expand ÷ 2 ÷ 2 6 − 1 = sequence or equation
5x – 15 = 4x + 8 3 + 7 = 7
-4x -4x 2 Inverse – to do the opposite action (for
x – 15 = 8
+15 +15 example adding is the inverse of
x = 23 subtracting)